A few months ago I was talking to you about the Magical Mathematics in our class. I would like to give you more information about these lessons. Many of the lessons I talked about, such as the Number Rods and the Golden Bead Decimal work, have several extension lessons that help sustain your child’s interest. This is accomplished by applying the information they retained from those first beginning exercises while being introduced to new activities using the same material.
Once the beginning information with the red and blue number rods has been well established through repetition we then give the child another opportunity to discover many mathematical facts. For example, if the child places the Number One Rod end to end with the Number Two Rod it will be exactly the same length as the Number Three Rod. The child is also able to see basic multiplication and division; for the Number Two Rod will fit on the Number Six Rod exactly three times.
The child can also use the rods to demonstrate the various combinations that equal the Number Ten Rod. He can place the Number One Rod beside the Number Nine Rod, the Number Two Rod beside the Number Eight Rod, the Number Seven Rod beside the Number Three Rod, The Number Six Rod beside the Number Four Rod and the Number Five Rod taken twice.
We can now introduce a new concept using a new material called the Spindle Boxes. The Spindle Boxes represent a parallel exercise in associating the numerals with the proper quantities. This time the numerals are in a fixed order and the quantities are loose using spindle rods to count. The Spindle Boxes have ten compartments labeled with the figures Zero through Nine. In a separate box, there are forty-five spindles. The child puts one spindle in the compartment labeled 1, two spindles in 2, etc. The first compartment is labeled 0 and this is the child’s first introduction to this symbol. He usually wants to put a spindle in this compartment but he must learn that Zero means none or nothing.
After the Spindle Boxes come the exercise called the Cards and Counters. In this exercise both the symbols and the quantities are loose and both must be placed in order by the child doing the exercise. First, he arranges the numerals in ascending order. When placing the appropriate number of red discs (counters) under each figure, the child puts the discs in rows of two. Each odd number has only one disc in the bottom row. This arrangement automatically illustrates the odd and even numbers.
There are a few other lessons that we introduce to the child as well such as the ten and teen boards but I will talk about that in a later blog. What I want to focus on right now are the Golden Bead Bank Games.
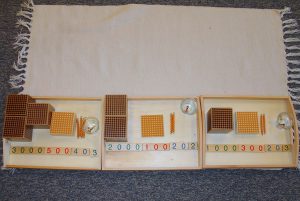
Any exercise involving the exchanging of the Golden Beads (or duplication of them) is usually called the Bank Game in the Primary class. The large quantity of material that the children use as a source for the game is referred to as the Bank. The children use the bank whenever they want to change Units to Tens, Tens to Hundreds, Hundreds to Thousands, or vice versa. Adding, subtracting, multiplying and dividing four place numbers can all be done with the quantities in the Bank.
If two children wish to add, each one puts a quantity of bead material on a small tray and selects the corresponding cards to represent the quantity. They then combine the two quantities on a larger tray and select larger cards to represent their total.
For subtraction, the teacher places a large quantity of bead material with the corresponding large cards on the large tray. She then gives the child a smaller tray with the number printed on a smaller card. The child “takes away” this quantity of bead materials from the larger tray and puts it with the smaller cards. The quantity remaining on the larger tray is the answer. The child then finds the numeral cards to represent this remainder. With this process the children learn that subtraction is the breaking up of one large quantity into two smaller ones.
When learning division, the child is taught that division means sharing and that the answer (quotient) is always what one person receives. If he has a problem 1294 divided by 3, he, serving as the Banker, asks three other children to get empty trays while he gets materials to represent the quantity 1294. He wants to share this amount equally among the three children, beginning with the One Thousand Cube.
Since he obviously cannot divide the single cube, he changes it at The Bank for 10 Hundred Squares. He now has 12 Hundred Squares—these 10 plus the original 2 in the number 1294—and he begins to share them among the 3 children. Each child receives 4 Hundred Squares. Next he divides the 9 Ten Bars; each child receives 3. Then he divides the 4 units; each child receives 1. One unit remains which he cannot divide. The answer is what one person receives, 431, with a remainder of 1.
I will continue our talk next time with the discussion about the several of the other Mathematical Materials we introduce to the older students as they move on from the concrete materials in the classroom towards the more abstract material which is the beginning of the memory work.